CRA Method: Concrete-Representational-Abstract–What Is It and How To Use It
What is CRA?
🔹 Concrete (C): Hands-On Learning
In the concrete stage, students engage in hands-on learning experiences using physical manipulatives. Picture students using base 10 blocks and a Place Value Chart to represent the number 126 or arranging bundled craft sticks to understand addition and subtraction. This tactile approach helps students develop a solid grasp of mathematical concepts by connecting abstract ideas to real-world experiences.

Moving on to the representational stage, students transition from physical manipulatives to visual representations. Here, they use drawings, diagrams, or other visual aids to represent or replace physical objects. For example, students might draw a base ten drawing of 126 to represent the quantity they previously counted with sticks. (Using a square to represent hundreds, tally marks for tens, and dots arranged in a dice pattern for ones.) Visual representations provide a bridge between concrete experiences and abstract concepts, helping students visualize mathematical relationships.
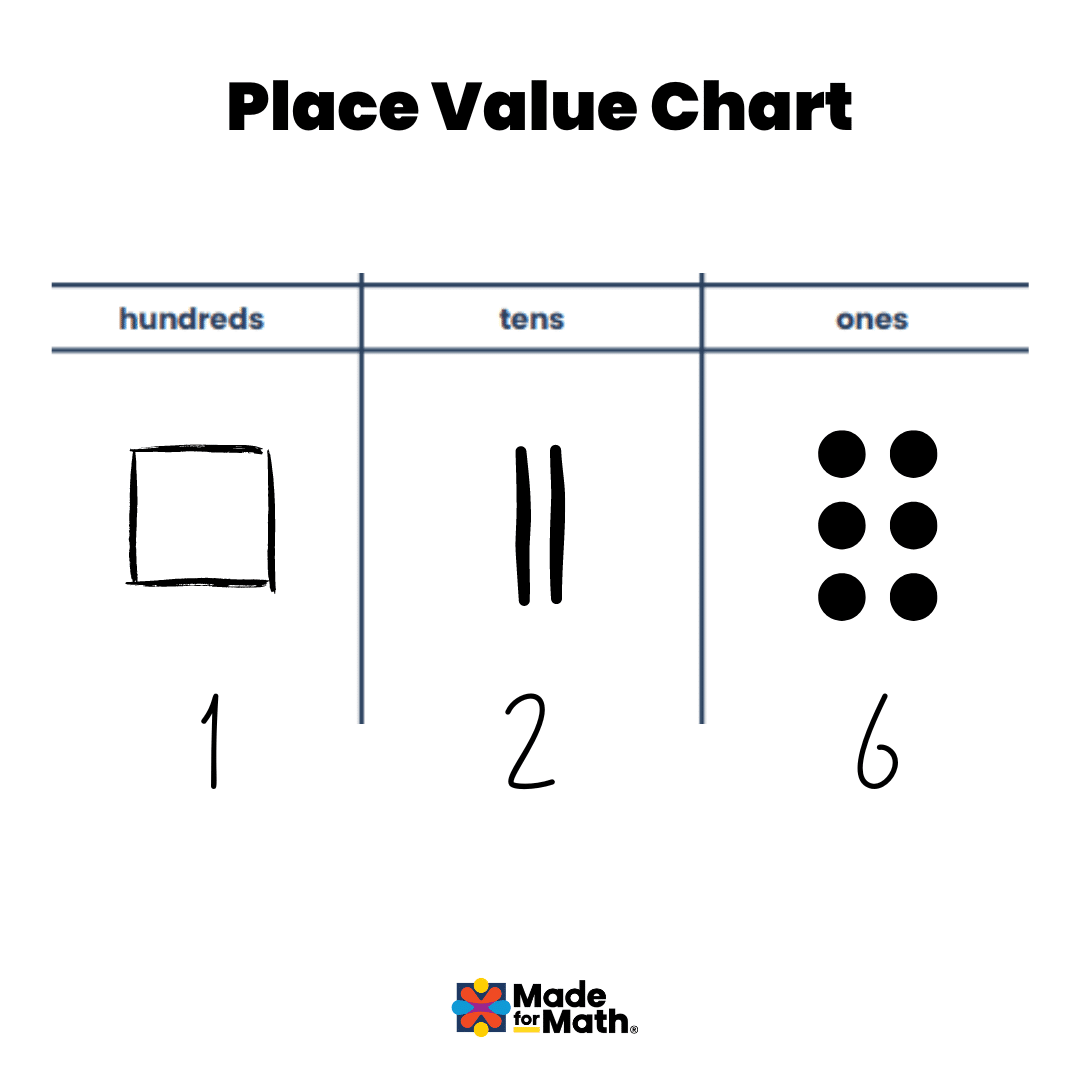
Finally, in the abstract stage, students work with numbers and symbols to represent mathematical concepts. This is where they understand that numbers and symbols can stand in for the concrete objects and visual representations they’ve encountered earlier. For instance, students recognize that the numerals 1, 2, and 6 in the number 126 represent a value of 1 hundred, 2 tens, and 6 ones. The abstract stage solidifies students’ understanding of mathematical concepts and prepares them for more complex problem-solving tasks.
The abstract stage includes standard algorithms, which are needed for efficiency and accuracy since drawing or building math problems every time would become time-consuming and error-prone.

CRA as a Framework–Not a Sequence
But here’s the thing – the CRA method isn’t just a linear progression like climbing a staircase. It’s more like a dynamic framework where students move fluidly between the concrete, representational, and abstract stages, making connections and building understanding along the way.
Research shows that both conceptual AND procedural knowledge are essential for math proficiency (Powell, 2022).
Since we are mapping students’ understanding of quantity to abstract mathematical symbols and numerals, concrete or pictorial representations should always be shown with the numbers and equations alongside, rather than the abstract phase of CRA only being experienced alone after concrete and representational work. Each stage in CRA supports the others.
Explicit Instruction
The CRA method works most effectively when paired with explicit instruction, especially for students with math learning disabilities.
For each stage, teachers should provide step-by-step instructions, modeling, and guided practice to help students grasp the underlying concepts.
As students progress to the abstract stage, explicit instruction plays a crucial role in building mastery of symbolic representations. Teachers provide explicit explanations of mathematical symbols and their corresponding meanings, guiding students in making connections between abstract symbols and concrete experiences.
Clear explanations, guided practice, and intentional connections ensure that students actively engage with math concepts and build a solid foundation for mathematical proficiency. By incorporating explicit instruction into each stage of the CRA method, educators create a supportive learning environment where students with math learning disabilities can thrive (Agrawal, 2016).
Want to see the CRA Method in action?
Resources
Are you still looking for more information? Here’s a few articles mentioned in this blog post and other relevant content.
Maths Practices You Can Count On: Five Research-Validated Practices in Mathematics by Sarah Powell
Myths That Undermine Maths Teaching by Sarah Powell, Corey Peltier
Check out our Interview with Corey Peltier here.
Evidence-Based Practices: Applications of Concrete Representational Abstract Framework across Math Concepts for Students with Mathematics Disabilities by Jugnu Agrawal and Lisa L. Morin
Are You Looking for a Better Way to Teach Math Facts?
Check Out Math Facts to Memory™ Vol 1 (DIGITAL ONLY)
Stop wasting your time with flashcards and rote memorization for students that learn differently. Discover the power of multisensory techniques in math fact instruction.
Over 40 lessons covering adding and subtracting within 10
- Explicit instruction for both YOU and the student
- Specific language to enhance learning
- Math vocabulary pages featuring morphology and gross motor movement
- Student practice sheets
- Games to practice math facts
Math Facts to Memory™ Volume 1 is appropriate for all learners but is essential for those with dyslexia and dyscalculia.
Grab your copy for just $35.
Updates for the life of the product!
MFM Authors

Jennie Miller
Marketing Assistant
is our Marketing Assistant and content creator here at Made for Math. Jennie loves being part of a company that is working to make mathematics accessible to children with dyscalculia.